Molecules have vibrational normal modes in addition to rotational and translational motion. Separation of translational motion from electronic motion can be done exactly as we discussed in the solution for the hydrogen atom. However, other types of motion cannot be separate exactly. Instead, we need to make an approximation in order to solve for electronic motion without considering the nuclei. The Born-Oppenheimer approximation permits the separation of variables based on the large difference in the mass. A proton has a mass 1860 times greater than an electron. Based on this difference we will assume that the time scale for nuclear motion is slow relative to electronic motion. This will be permit us to write an equation for nuclear motion that is separate from electronic motion. We have already solved an equation for nuclear motion using the harmonic approximation for vibrations and the rigid rotator for rotations. The electronic part can then be treated using a method that involves a linear combinatino of atomic orbitals.
The exact solution for the hydrogen molecule ion
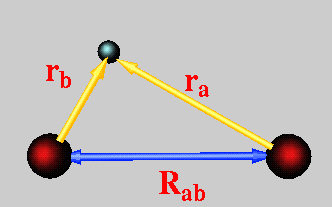
The Born-Oppenheimer approximation can be implemented in the simplest possible molecule, the hydrogen molecule ion. This molecule has only one electron that is delocalized over two nuclei. Because there is only one electron the hydrogen molecule ion can be solved exactly.
The variational principle
The wave equations can be applied to the hydrogen atom. Using spherical polar coordinates there is an exact solution. The wave functions for the hydrogen atom are the most important solutions in all of quantum chemistry. The shapes of the angular part, which arethe familiar s, p, d and f orbitals form the basis for approximate methods that permit calculation of the energy and other properties of atoms and molecules. THe hydrogen atom is the only atom, for which there is an exact solution, but that solution is the starting point for all approximate solutions. The energies of the hydrogen atom agree with experiment and are equal to he result obtained by Bohr using semi-classical methods.
The wave equation in quantum mechanics
- There is no ballistic motion in quantum mechanics since particles behave like waves and therefore the equation of motion is a wave equation.
- Instead of defining precisely where particles are located in space (i.e. using coordinates x, y and z), the solution of the wave equation is a wave function that can be used to calculate the probability for the particle to be in a given region of space.
- The probability can be calculated from the square of the wave function. The square will involve a product of the complex conjugate if the wave function is complex. The wave function must be normalized in order for the probability to be meaningful.
- The wave function can also give properties of the wave function solution in the form of operator equations. For example, the energy can be calculated using an energy operator, known as the hamiltonian. The momentum can be calculated using a momentum operator, and so on.
|