Studies of the photosynthetic reaction center

The photosynthetic reaction center is the protein-pigment complex that converts light energy from the sun into chemical energy in plants and bacteria. The structure of the bacterial photosynthetic reaction center was solved by X-ray crystallography, and represents the first membrane protein structure that was determined. This structure and the organization of the chromophores is shown in Figure 1. The protein spans the periplasmic membrane and when combined with the bc1 complex constitutes a light driven proton pump in the bacterium. The coupling of light energy to proton uptake and translocation occurs involves the cofactors shown in Figure 1B. These are the primary donor, P, the primary acceptor H, the secondary acceptor Q_A and finally the removable quinone Q_B. When the primary donor of photoexcited to form a singlet state ¹P,

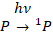
(1)

it can rapidly transfer an electron to the bacteriopheophytin, H, to create the charge separated state P⁺H^- (t ~ 1 ps).

(2)

A bacteriopheophytin (BPheo) is structurally the same as a BChl except that it lacks the central Mg atom. Subsequently, in a slower step the electron can be further transferred to the quinone Q_A to create P⁺HQ_A^{- (}t ~ 100 ps).

(3)

If Q_B is present (and it may not be since it can leave the RC) the electron will transfer on to Q_B to create P⁺Q_B^{- (}t ~ 100 ms).

(4)

P⁺Q_B^- can take up a proton to make P⁺Q_B^- + H⁺ à P⁺Q_BH. This form of the RC is inactive, but it is reduced by a cytochrome c molecule P⁺Q_BH + Cyt c à PQ_BH + Cyt c⁺. Now, the entire series of electron transfers can proceed again to create a second P⁺Q_BH^- , which can take up a second proton

(5)

Finally, can leave the RC and migrate to the bc1 complex where its cargo of 2H⁺ is given the proton pumping complex. The essence of the RC’s function is to convert light energy into electrical energy that can be used to capture and translocate protons.

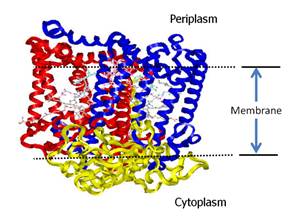
Figure 1. Structure of the bacterial photosynthetic reaction center (RC) from Rhodopseudomonas sphaeroides.

From a fundamental biophysical perspective, the electron transfer reaction is the most basic type of process. There are at least three electron transfer reactions that are important in the normal function of the RC. Figure 1B shows that there can be short circuit back to the starting point if Q_B is not present. The charge combination reaction,

(6)

Does not occur if Q_B is present, but will occur in an isolated RC is Q_B is removed. This state of the RC is useful since it can be relatively rapidly reset (t ~ 100 ms). Just like the example of photolysis of myoglobin, the RC can be studied by laser photolysis in a manner that permits signal averaging. The rate scheme that describes the cycle of electron transfers is shown in Figure 2.

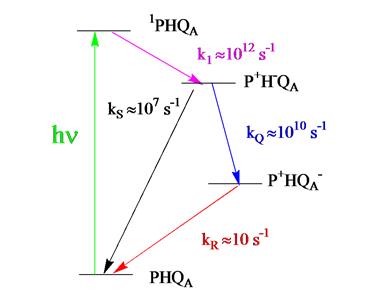
Figure 2 Kinetic scheme for the photosynthetic RC.

Electron transfer theory

Electron transfer (ET) reactions are the fundamental kind of chemical process that can be studied from a theoretical point of view. The quantum mechanical treatment of ET has both an electron and a nuclear part, just like absorption of light. However, unlike absorption of light, there is no input of energy in electron transfer, so the initial state must be higher in energy than the final state. We call the energy difference between the states, e, which is really an enthalpy, DH^o for the reaction. In fact, the entropy, DH^o, can be included in the calculation so that e actually represents the free energy, DG^o. Figure 3 shows that there is a barrier between the reactants (shown in blue) and products (shown in red). For example, for a charge separation reaction the reactants are DA (donor-acceptor) and the products consist of D⁺A^-. The barrier is determined by the relationship between the energy gap, e and the reorganization energy, l. The reorganization energy is equivalent to the energy required to distort the system from the final geometry to the initial geometry along the reactants potential surface. We can relate the barrier height e* to the energy gap and reorganization energy.

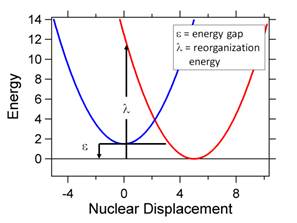
Figure 3. A. The energy gap, e, and reorganization energy, l, are defined using the potential energy surfaces of the reactants (blue) and products (red) as a reference. B. Three different cases with different geometries are shown for the reactant and product surfaces. Depending on the relative values of e and l, the activation energy, e*, can be zero (activationless) or positive (either e < l or e > l).

The energy barrier, , which is also the activation energy, is derived in Appendix B.

(7)

Eqn. 7 relates the fundamental parameters of Marcus theory, the energy, e and reorganization energy, l, to the activation energy. If we consider a classical theory such as the Arrhenius theory for the rate constant we would have,

(8)

If we consider the quantum mechanical model for the rate constant,

we can write

(9)

where the normalized Gaussian,

(10)

is the Franck-Condon factor. The functional form of the ET rate constant has an electronic part, which is related to the overlap of the donor and acceptor wave functions, and a nuclear part, which depends on how closely matched e and l are. The electronic part has an exponential dependence on distance, which comes from the exponential decay of the wave functions as represented in Figure 4. is the non-radiative analog of , the square of the transition moment. For light-driven processes the coupling is induced by the radiation field. For electron transfer (atom transfer etc.) the coupling is proportional to the overlap of the donor and acceptor wave functions. Speaking more precisely, the overlap is proportional to the reactant and product wave functions, but we can consider, for example, the overlap of the HOMO of the donor, D, and LUMO of the acceptor, A, in a charge separation process

Since effectively that is the overlap of with . This is illustrated schematically in Figure 4, from which it is clear that the overlap decreases exponentially with distance. Thus, we can write,

Where R is the internuclear distance and depends on the details of the medium between the donor and acceptor. As general rule it is thought that

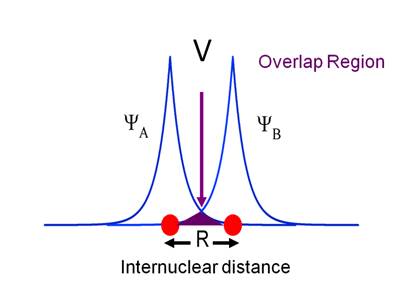
Figure 4 Depiction of the overlap of electron wave functions that leads to electronic coupling.

Figure 3B shows that the different barrier heights or activation energies, , as a function of e and l. When e = l, then = 0, and the rate is maximum. This regime is called activationless. It is clearly desirable for forward electron transfers. However, the Marcus equation also shows us how the RC can prevent undesirable charge recombination from occurring. This can be accomplished by detuning e and l, so that is large for reverse electron transfer.

The quantum yield for photosynthetic electron transfer

For each step in a series of electron transfer reactions, there is a quantum yield for the forward (productive) electron transfer process,

where k_f is the forward rate constant and k_r is the reverse ET rate constant. The objective of efficient capture of solar energy will be achieved if this quantum yield can be maximized.

We can examine the example of the reaction

Where k_Q is the productive forward electron transfer that creates the state and k_S is an undesirable back electron transfer that wastes the light energy by returning to the original state without doing any useful work. In this case, it is relatively straightforward to explain the efficiency of the forward charge separation process relative to the reverse reaction that leads to the ground state. We need to have an estimate of the reorganization energy for these processes. The reorganization energy, l, is a measure of the displacement of the potential surface of the excited state. In fact, we can relate it to S and say that

For one mode or

If many modes are coupled to the electron transfer process. It is the reorganization of the protein around the active donors and acceptors in Figure 1 that contribute here. Experiments have shown that the forward process is optimized, which means that it is near the peak of the Marcus curve shown in Figure 5. The driving force is, e = 4000 cm^-1 and the reorganization is nearly perfectly matched at l = 4000 cm^-1. Thus, e* 0 for this process. Given that the rate constant is approximately 10¹⁰ s^-1, we can calculate that

as shown in Appendix C. Thus, the forward reaction is activationless and has significant electronic coupling. This idea of a maximum rate constant is shown in Figure 5. Figure 5 also shows that the the rate constant decreases when e > l. This is known as the inverted region.

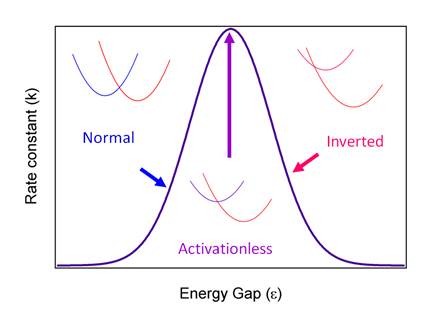
Figure 5. Plot of the Marcus theory regimes of normal, activationless and inverted.

For many years scientists tried to prove that the inverted region existed. In fact, there is now little doubt that the rate constant decreases as the driving force increases. After many years Marcus wrote a paper pointing out the plot in Figure 5 looks like an absorption spectrum. Since the Hamiltonian is not an interaction with radiation, but rather a non-radiative process involving coupling of states through overlap, the electronic parts differ, but the nuclear parts are the same. You should think of Figure 5 as a “spectrum”, but it is the non-radiative “spectrum”. Any given molecule can have only one e and one l, and those values determine where the molecule resides on the curve in Figure 5. We now turn our attention to the electron transfer reactions in bacterial photosynthesis shown in Figure 1.

The distance from H to Q_A is actually closer than the distance from H to the special pair, P. However, we know that P is strongly coupled to H because of the very efficiency forward process. For our simply consideration of how the protein may control the electron transfer process we will simply assume that the two electronic couplings are about the same

Given this simple assumption we might expect that the rate constants would similar in magnitude. If this were the case the quantum yield for forward electron transfer would around 0.5, which is not very good for efficient charge separation. The point here is that control over charge separation efficiency can be made at the level of the FC factor. In this case, the driving force for back electron transfer is large, e 8000 cm^-1. While we might expect that large driving force to result in a fact rate (larger rate constant), we must remember that the Marcus theory shows that large driving forces actually reduce the FC factor since they are no longer activationless. The increase in e does not mean that l is larger too, and in fact all of the evidence would suggest that l is smaller for the reverse electron transfer process. However, once again to simplify the analysis we will assume,

Thus, here the reverse electron transfer process, , has

Here we see that the activation energy is significant, and therefore the FC factor will be much smaller for K_S then for k_Q. Finally, we calculate the quantum yield for the forward process, k_Q shown in Figure 2,

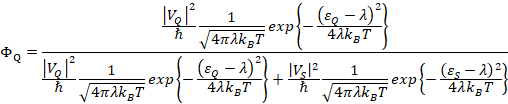
But, of course, the prefactors are all equal by our simplifying assumptions. Moreover, e_Q* = 0. Therefore, the cancellations yield,

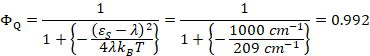
The quantum yield for forward electron transfer to the quinone, Q_A is greater than 99%. The control of the direction of the flow of electrons occurs mainly because of the balance of nuclear reorganization and driving force (free energy change) of the electron transfer reactions.

The quantum yield for the primary charge separation step

Although the primary charge separation step is the most important process in the RC, it is also the most difficult to understand and to rationalize. The light energy captured by the special pair of bacteriochlorophylls (BChls), known as P, is converted into the excited state in about 1 ps at cryogenic temperature (even < 4K)! In fact, the rate constant for the process increases with temperature. This increase is predicted by Marcus theory. An activationless process, e* = 0 has only the prefactor and so it is predicted to depend on temperature as,

It turns out that this is not really the hard part to understand. Rather it is the high quantum yield and directionality of the charge transfer that has caused many years of research. The process and rate scheme are shown in Figure 1.

The driving force for the primary charge separation is very small. It is estimated to be about e = 1000 cm^-1. Given the preceding discussion we would expect that the rate would not be at the maximum of the Marcus curve, unless l = 1000 cm^-1 as well. We saw in the previous section that l = 4000 cm-1 is a more typical number for an electron transfer reaction in a protein. In aqueous solution where water molecules can reorient around the charges, even larger values are observed (e.g. l = 8000 cm^-1). Somehow, the special pair permits electron transfer to occur in a concerted manner that has very low effective solvent reorganization energy. Perhaps part of the trick lies in the absorption process itself.

When P is excited it has a large excited state dipole moment. This is created instantly and is inherent in the absorption process. The dipole moment of P must actually contain a significant reorganization, but this reorganization is “paid for” by the light energy and not by the transferring electron. You can think of this as the creation of some amount of during absorption. This partial charge separation “pushes” the electron along the reaction coordinate that leads to. At this you might think that the best way to capture light energy, and move an electron would be to go all the way and make a charge transfer absorption process. P stops short of this. The reason is that charge transfer absorption bands have rapid relaxation that leads to rapid dephasing and non-radiative decay back to the ground state. As Figure 6 shows, the non-radiative decay within P can also reduce the quantum yield. Thus, P is a finely tuned electron transfer machine that has just enough charge separation to push the electron on the path towards H, but not so much that it destroys the efficiency of charge separation.

We can prove this by comparing the efficiency of P, a dimer, with the heterodimer mutant. In the heterodimer, shown in Figure 6, one of the Mg atoms of the chlorophyll does not insert during folding. Thus, instead of P with two BChls, the RC has b, which has one BChl and BPheo. The consequence of this is that the absorption band is almost a pure charge transfer band, . The consequence is that non-radiative decay is much greater and electron transfer slows down as well. The net effect is that the quantum yield for primary separation drops from nearly 100% to 50%.

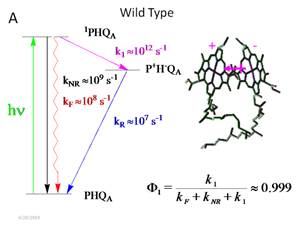
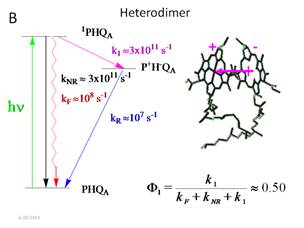
Figure 6. Kinetic scheme for the primary charge separation reaction.

The unique absorption of light energy in the photosynthetic RC

In the previous section we have suggested that a dimer of BChl molecules has the ability to absorb light and create a partial charge separated state. This has been measured by electric field effect spectroscopy. If assume that the dipole moment in the ground state is 0 by symmetry (see Figure 1, which shows the symmetry of the RC), we can understand that there is symmetry breaking in the excited state if the state is created so that the electron is one side of the RC. The measurement suggests that the dipole moment in the excited state

is ~7 Debye. This relatively large dipole moment indicates that the special pair has partial charge separation. The coordinate s can be x, y, or z and depends on the coordinate system.

This large charge separation implies that the nuclei have a large displacement in the excited state. This can be understood since large charge displacement requires a significant change in the bonding electronic structure. The nuclear displacement along certain key vibrational modes that involve the dimer should be significant. These displacements have been measured by Raman scattering. The result is shown in Figure 7. The net effect is that the electron-vibration coupling, S, is large for P relative to B or H. This can actually be seen directly in the absorption spectrum, also shown in Figure 7. The absorption band for P is shifted and broadened, which indicates that it has a different FC progression. Quantitative information on the magnitude of the shift in the excited state surface can be obtained from the intensity of the Raman bands. Figure 7 shows that there are several low frequency Raman bands observed when the excitation laser is tuned into resonance with P, that are not observed when the laser is in resonance with the BChl monomers, B.

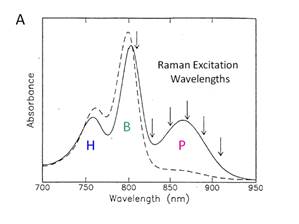
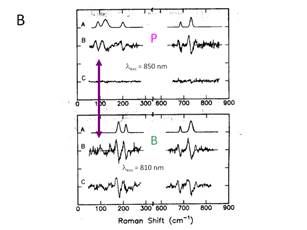
Figure 7. Absorption and resonance Raman data for the chromophores of the bacterial RC isolated from Rsp. Sphaeroides. A. The absorption spectra for the three chromophores, the BChl dimer P, the two BChl monomers, B, and the BPheo, H are shown. B. The resonance Raman spectra obtained using a special method to observe small Raman bands on a large fluorescent background is shown.

The FC progression depends on the form of the nuclear overlap factors, which we have seen is

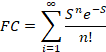
For a single mode. Suppose that many modes are coupled to a reaction. Then the FC factor is the product of those single mode factors, i.e.

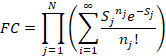
where each mode has its own electron vibration coupling constant S_j. The Raman results suggested that for P, there is an important 30 cm^-1 vibrational mode with a large S_j. This mode could be an intradimer stretching mode. Obviously, this mode is absent in a monomer. This coupling may lead to the observed absorption band of P and this may in turn be connected to the excited state nuclear displacement observed by electric field effect spectroscopy. These speculations are supported by a number of experimental observables that are beyond the scope of this course. However, the example of photosynthesis provides a window into the manner that biophysics is applied to the study of important biological problems such as the capture of the Sun’s energy by plants and bacteria, which is the origin of all life on the planet.